Why Is Quantum Mechanics So Difficult To Understand?

Quantum mechanics is difficult to understand because it is based on postulates about wave functions that are difficult to relate to. Moreover, quantum particles do not obey classical laws, making it difficult to predict their behavior. Additionally, the mathematical framework of quantum mechanics is difficult to understand.

If you have ever read sci-fi comics, you must have noticed some of the buzzwords that get thrown around quite often. Words like “quantum mechanics”, “singularity”, “quantum decoherence” and whatnot. They seem to catch readers’ attention unlike anything else and introduce a sense of mystery regarding the phenomena, making the reader wonder about the quantum world. This contributes to the narrative that QM is “hard” to understand. 

Well, let’s attempt to demystify the quantum world, for the true beauty of science lies not in the wonder of mysterious phenomena, but in the repeated attempts at understanding them and pushing the boundaries of knowledge. 

Quantization Of Energy: Breaking Established Laws

The word ‘quantum’ in physics is not an adjective that would describe a certain entity. Instead, quantum actually comes from the word ‘quantized’. In the 1900s, it was discovered that the energy of micro-scale systems (a collection of molecules, atoms, electrons bound to a metal, etc.) can only have certain allowed values.

Imagine throwing a tennis ball. The speed of the ball could, in principle, have any speed in the continuous range from 0 km/h to 150 km/h (realistically, based on arm strength). However, if the ball were subjected to quantum mechanical laws, then the ball would move only at certain allowed values, for example, at speeds that are multiples of 2km/h (i.e. 2, 4, 6, 8, …) or at speeds that are exponents of 3km/h (i.e. 3, 9, 27, 81,…). This would mean that there exist some forbidden speeds at which the ball cannot move. Thus, the plot of speed versus time would be a discontinuous plot. This rather absurd phenomenon is actually true for quantum particles like electrons and protons. This is called the quantization of velocity. Since kinetic energy depends on velocity, the quantization of velocity also leads to quantization of energy.

Ultraviolet Catastrophe: First Hint Of The Quantum World

In the late 19^th century, physicists tried to calculate the total energy emitted by an ideal blackbody. This involved summing the energies of all the atoms constituting the blackbody using the laws of classical physics. What followed shook the foundations of physics to its core. It was calculated that the energy emitted by a blackbody in thermal equilibrium with its surroundings is infinite. In layman’s terms, this implied that the energy emitted by the blackbody is greater than the energy of all the stars, planets, comets, asteroids, etc. in the universe combined.  

This absurd result prompted physicists to look for alternative theories. The problem was solved when Max Planck assumed that the atoms making up the blackbody emitted energy with only certain allowed values. In simple terms, it meant that energy emitted by all the atoms was quantized. Simplifying this further, each atom could emit certain allowed energies. This simple assumption solved the problem of infinite energy and theoretical calculations (which earlier gave absurd results) matched with practical observation. 

Also Read: What Is Quantum Physics?

Weird Phenomena At The Quantum Scale

What if you were told that you have a wave associated with you? Well, believe it or not, so does your car, your house, and your clothes. In fact, all physical matter in the universe has a wave nature associated with it. 

Single Electron Interference

Interference is the superposition (vector addition) of waves of the same frequency traveling in the same direction.

In this experiment, a source emits electrons towards a photographic film that is kept some distance apart. Between the source (S) and the film (F) lies a wall (W) with two holes in it, also some distance apart. The electron emission rate is controlled such that only a single electron crosses W at a time. A classical analogy would be a tennis ball thrower shooting towards a wall with two holes such that at any time, only a single ball crosses the wall. A sheet behind the wall records the area where the ball hits.

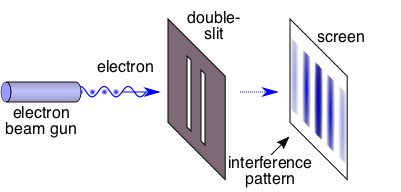
Classical theory (tennis ball) would predict that the striking pattern obtained on the sheet would be random. Extending this theory to the quantum world (single electron), a random strike pattern on the photographic film (F) would be expected. 

However, the observations surprised physicists. The pattern obtained on F consisted of areas of high intensity and low intensity placed a regular distance apart. This pattern is similar to an interference pattern caused by light waves. After identifying a clear pattern characteristic of waves being caused instead by particles (electrons), it was concluded that particles also have a wave-like character.

Quantum Decoherence: Destroying Observability

Continuing further with the single electron interference experiment, originally, the apparatus was kept out of observation while it ran. It means that while the electrons were being emitted and hitting the film F, no observation of the process was made. After the experiment ran for a few hours, it was stopped and the film F was retrieved. However, what if the experiment was recorded by a camera? Or observed with the naked eye?

Well, quite surprisingly, the interference pattern vanished, or rather, no pattern like the earlier one was observed. In this case, the pattern was random, just like the tennis ball experiment. In other words, observation destroys interference. This isn’t the case with tennis balls though. You may keep as many cameras and spectators present as you want, yet the pattern wouldn’t change.   

This happens because, at the quantum scale when light photons strike the electron, the electron is disturbed from its original path. Thus, in an attempt to make a measurement, pure observation is lost. 

Quantum Tunnelling

Imagine that a tennis ball was restricted to move in a room with infinitely high walls. There’s no way that the ball would cross through the walls, even at high speeds (no breaking of the wall is allowed). Because crossing the wall requires the ball to be higher than the wall height, it is actually impossible for the ball to move out. Similarly, on the quantum scale, if an electron is restricted to an infinitely deep potential well (akin to walls), one might expect the electron to remain trapped forever with zero chances (read probability) of crossing the wall. However, QM is here to surprise us again. There does exist a finite probability that the electron will cross the potential wall when it strikes. This is observed even in real life. Two metallic conductors are soldered together to immobilize a joint. Yet, the solder is only a potential barrier. Electrons have a finite probability of crossing the wall and thus the circuit remains closed and electricity flows.   

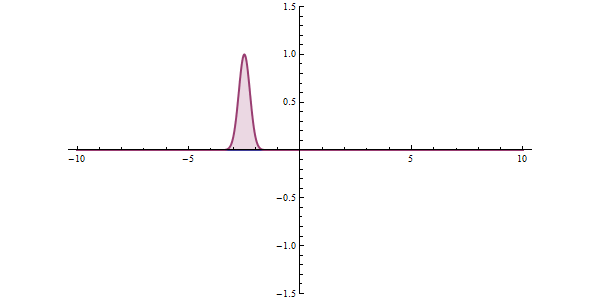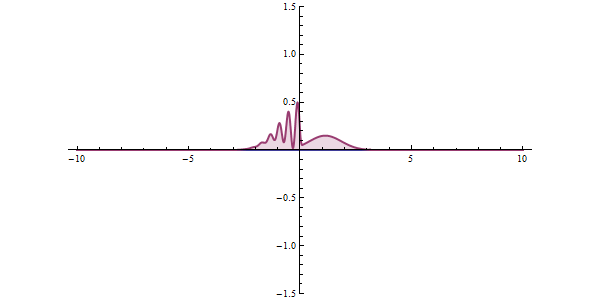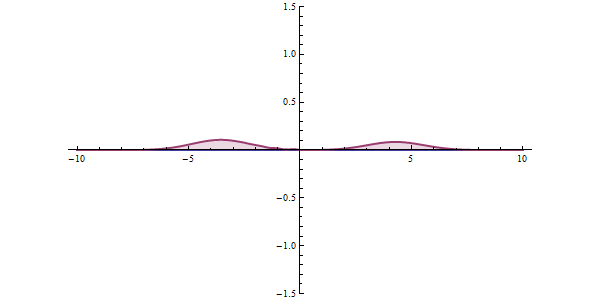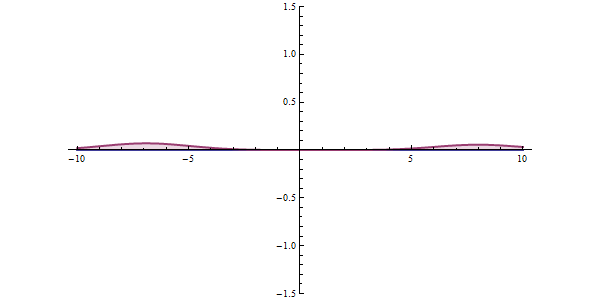
Also Read: Without Magic, Could You Get Through Platform 9 ¾?

Conditioned Thinking With Classical Physics

Since introductory and intermediate-level physics involves classical physics, it is easier to relate to daily phenomena using these theories. However, when quantum mechanics is first introduced, it invariably starts with postulates about wave function that are difficult to relate to. Moreover, since quantum particles don’t obey classical laws, predicting their behavior becomes difficult, as does understanding the mathematical framework of QM. It’s not easy to think about operators and wave functions without trying to find a classical analogy… which simply doesn’t exist!

Also Read: What Is Quantum Mechanics?

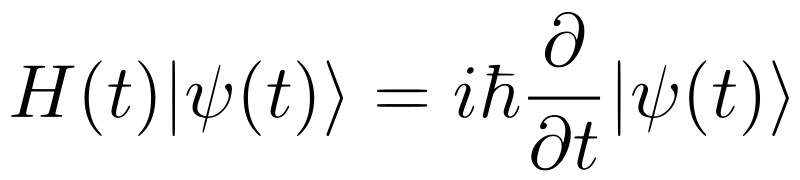
How well do you understand the article above!

Can you answer a few questions based on the article you just read?

Start Quiz

Question

Your answer:

Correct answer:

Next

You got {{SCORE_CORRECT}} out of {{SCORE_TOTAL}}

SHARE YOUR RESULTS

ShareTweetPinEmail

Your Answers

Retake Quiz